BlueGas
Well-Known Member
- Joined
- Sep 20, 2014
- Messages
- 2,439
- Gender
- Male
- HSC
- N/A
I need help for this question even though it may seem easy.
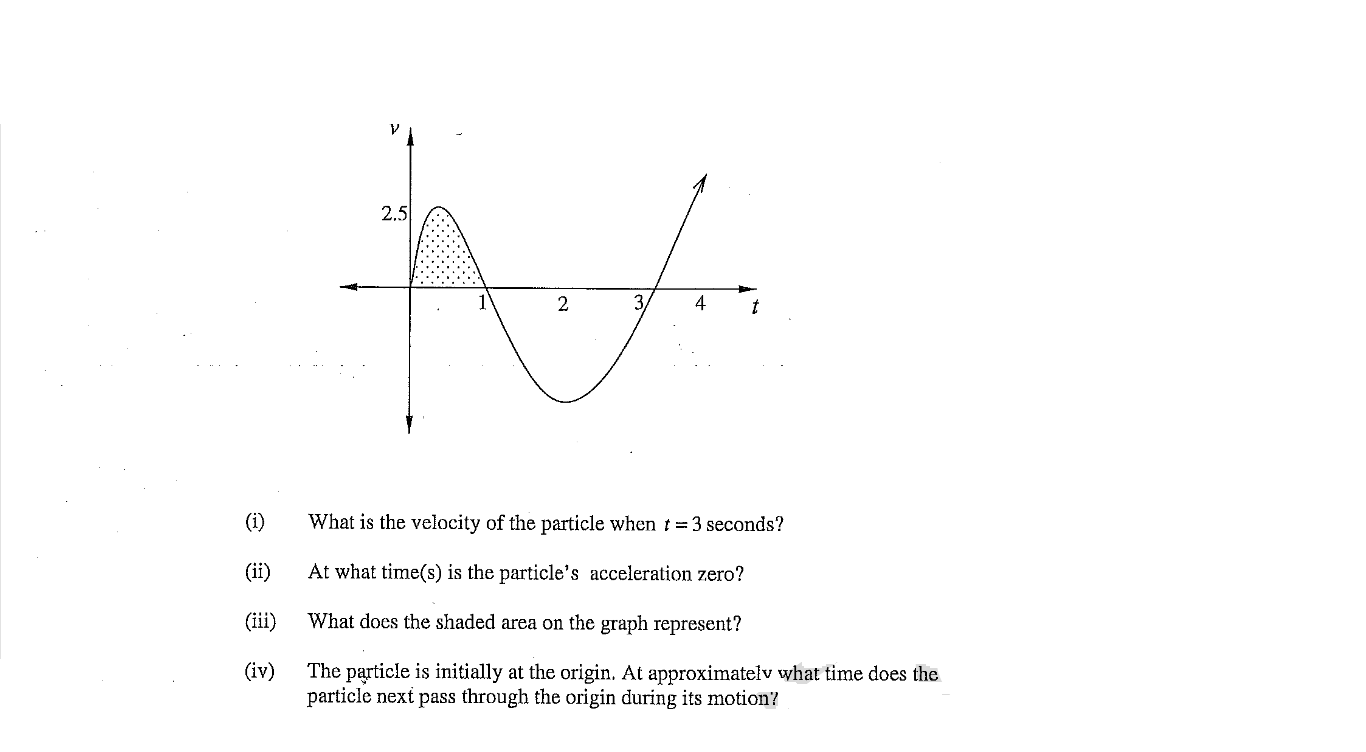
i), v = 0 when t = 3
ii) Basically I should be looking for the times when f''(x) = 0, or when it's a point of inflexion, right?
But the answer says t = 1/2 and t = 2, and these are stationary points, but that's when f'(x) = 0, I need some clarifying here.
iii) Shaded area represents distance travelled in the first second
iv) The answer is apparently t = 2, but how? From what I know, a particle reaches the origin when it hits the t-axis. I also need clarifying here because I may be wrong.

i), v = 0 when t = 3
ii) Basically I should be looking for the times when f''(x) = 0, or when it's a point of inflexion, right?
But the answer says t = 1/2 and t = 2, and these are stationary points, but that's when f'(x) = 0, I need some clarifying here.
iii) Shaded area represents distance travelled in the first second
iv) The answer is apparently t = 2, but how? From what I know, a particle reaches the origin when it hits the t-axis. I also need clarifying here because I may be wrong.
