Maths Help Please (1 Viewer)

Jojofelyx · Jul 29, 2021

Could someone please explain how these are solved? Thank youuuuu!

notme123 · Jul 29, 2021

I think that the last one is a 4u question only but used to be 3u.
7)

Nevertheless, the first one I think might be C.
This is because we see a double root at x=-2 for f'(x). That means f(x) must have x=-2 as a triple root. This means $(x+2)^3$ is a factor. Hence A and B are eliminated. From here, it's obvious it's C. f'(x) never hits the x-axis again as x approaches negative infinity, meaning f(x) has no turning points back there, hence it cant have another root below 0. Similarly, it cant have a root between 0 and 4 because there are no turning points there either. Therefore, it must have a root at x=6, meaning (x-6) is a factor. Hence, the only possible roots in the options available that f(x) could have is C.

8)

Next one I prefer using implicit differentiation.
Basically, just differentiate everything in terms of x. Note the ys become y' times their differential. The first term is a product, so you use the product rule. $2(y(1) + x\frac{dy}{dx}) - 2x + 4y^4\frac{dy}{dx} + 0 = 0$
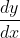
Make the $\frac{dy}{dx}$ the subject and simplify, you'll get $\frac{dy}{dx}=\frac{x-y}{x+2y^4}$
Sub in the point you get $\frac{dy}{dx} = \frac{-1-0}{-1+0} =1$

So the gradient is 1.
Point-gradient formula:
$y-0=1(x-1)$
$y=x-1$

So answer is B.
Please correct me if I got it wrong.

9)

For the last one, straight away I would sub in the initial condition for all options.
Doing this will eliminate C and D instantly because neither $v^2 = 7$ nor $v^2 =-4$ is correct.
Next easiest thing to do is employ the formula: $\frac{d}{dx}(\frac{1}{2}v^2) = a$
It's a bit of a pain but the easier one to do imo is A. If you dont know the method, square the equation, halve, then differentiare W.R.T x. Doing this youll get a = 8 which is wrong so it must be B.

CM_Tutor · Jul 30, 2021

notme123 said:
9)

For the last one, straight away I would sub in the initial condition for all options.
Doing this will eliminate C and D instantly because neither $v^2 = 7$ nor $v^2 =-4$ is correct.
Next easiest thing to do is employ the formula: $\frac{d}{dx}(\frac{1}{2}v^2) = a$
It's a bit of a pain but the easier one to do imo is B. If you dont know the method, square the equation, halve, then differentiare W.R.T x. Doing this youll get a = 8 which is wrong so it must be A.

I agree that using the initial position information to eliminate (C) and (D) is the first step.

There is an easier way for the last part, though, by using

$\ddot{x} = \cfrac{d}{dx}\left(\cfrac{1}{2}v^2\right) = v\frac{dv}{dx}$

For (A), we have

$\begin{align*} v &= 2 + 4\ln{x} \\ \cfrac{dv}{dx} &= 0 + 4 \times \cfrac{1}{x} = \cfrac{4}{x} \\ \ddot{x} &= v\cfrac{dv}{dx} = \left(2 + 4\ln{x}\right) \times \cfrac{4}{x} = \cfrac{8(1 + 2\ln{x})}{x} \\ \text{Put $x = 1$:} \quad a &= \cfrac{8(1 + 2\ln{1})}{1} = \cfrac{8(1 + 2 \times 0)}{1} = 8 \end{align*}$

For (B), we have

$\begin{align*} v &= 2\sin{(x - 1)} + 2 \\ \cfrac{dv}{dx} &= 2\cos{(x - 1)} \times 1 + 0 = 2\cos{(x - 1)} \\ \ddot{x} &= v\cfrac{dv}{dx} = \left[2\sin{(x - 1)} + 2\right] \times 2\cos{(x - 1)} = 4\cos{(x - 1)}\left[1 + \sin{(x - 1)}\right] \\ \text{Put $x = 1$:} \quad a &= 4\cos{(1 - 1)}\left[1 + \sin{(1 - 1)}\right] = 4\cos{0}\left[1 + \sin{0}\right] = 4 \times 1(1 + 0) = 4 \end{align*}$

As it happens, (C) and (D) allow straight-forward determination of $\ddot{x}$ using the other form stated:

For (C), we have

$\begin{align*} v^2 &= 4\left(x^2 - 2\right) \\ \cfrac{1}{2}v^2 &= 2\left(x^2 - 2\right) \\ \ddot{x} &= \cfrac{d}{dx}\left(\cfrac{1}{2}v^2\right) = \cfrac{d}{dx}\left(2x^2 - 4\right) = 2(2x - 0) = 4x \\ \text{Put $x = 1$:} \quad a &= 4(1) = 4 \end{align*}$

For (D), we have

$\begin{align*} v^2 &= x^2 + 2x + 4 \\ \cfrac{1}{2}v^2 &= \cfrac{x^2}{2} + x + 2 \\ \ddot{x} &= \cfrac{d}{dx}\left(\cfrac{1}{2}v^2\right) = \cfrac{d}{dx}\left(\cfrac{x^2}{2} + x + 2\right) = \cfrac{2x}{2} + 1 + 0 = x + 1 \\ \text{Put $x = 1$:} \quad a &= 1 + 1 = 2 \end{align*}$

So, the calculus would reduce the answer to (B) or (C) and (C) would still need to be eliminated on the grounds that it does not satisfy the initial conditions.

ExtremelyBoredUser · Jul 30, 2021

Responding to the 2nd question:

I think the whole implicit differentiation process is right but the substitution of the x value did not consider the -ve sign in front in the gradient intercept formula. Here is my working out just for reference? I could be making a mistake since I have not done implicit differentiation as much.

Maths Help Please (1 Viewer)

Jojofelyx

Well-Known Member

Attachments

notme123

Well-Known Member

CM_Tutor

Moderator

ExtremelyBoredUser

Bored Uni Student

Users Who Are Viewing This Thread (Users: 0, Guests: 1)