find the values for K for which L is a tangent to C
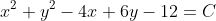
 that is the equation for line L
that is the equation for line L
i know that C is a cricle with radius 5 and centre (2,-3)
and the perpendicular length from centre of circle to line L is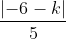
i know that C is a cricle with radius 5 and centre (2,-3)
and the perpendicular length from centre of circle to line L is
