Since the angle sum of a triangle is 180 degrees
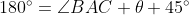
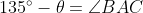
Using the sine rule:
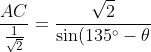} )
Since this is Extension 1, we can expand the sine function
 \cos \theta - \sin \theta \cos(135^{\circ})} )
Now:
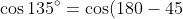^{\circ} = -\cos 45^{\circ} = \frac{-1}{\sqrt{2}} )
Angle in second quadrant, cosine negative.
^{\circ} = \sin 45^{\circ} )
Angle in second quadrant, sine positive.
Sub this back in:
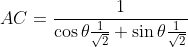
Multiply both top and bottom by the square root of 2.
