a)Calculate the area bounded by y=e^x, the coordinate axes and the tangent at x = 2
b) Calculate the area bounded by y=e^x, the Y axis and the line y=e^2
(note: for these problems drawing a diagram is absolutely essential!)
Lets find the equation of the tangent at x = 2
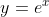


So the gradient of the tangent is
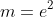
and the tangent goes through the point
)
So by the point gradient formula
)
)

To find the area enclosed between the tangent, the curve and the axes we find the area under the curve, ie y = e^x, then subtract the area under the tangent. So:
 dx)
Note that we are taking the integral between 1 and 2 for the tangent because the tangent is below the x-axis between 0 and 1 and so that area would be negative (which we don't want). Proceeding,

^2}{2} - 2e^2 - \left(\frac{e^2\cdot (1)^2}{2} - e^2\right)\right] )

 units^2})
(ii) Note that if we were to find the area bounded by the y-axis we would have to change y = e^x in terms of y instead of x, the result being x = log
e y. But you cannot integrate log
e y.
Use the graph you constructed in the last question. We know that y = e^2 when x=2. Draw both the lines y = e^2 and x = 2. Note that this forms a rectangle. We can find the area under the curve y = e^x between 0 and 2, then subtract that from the rectangles area which would give us the area bounded by the y-axis. So, the area under the curve y = e^x between x =0 and x = 2 is:


The area of a rectangle is length times width, ie

And so the area bounded by the y-axis is:
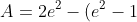)

 units^2})