Re: HSC 2017 MX2 Marathon ADVANCED
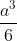 .
.
Consider a cube with side length a. If you cut along its diagonals u get 6 identical pyramids with height half the base.
It's justA square pyramid has height exactly half it's base side length, a. Find the volume of the pyramid.
Consider a cube with side length a. If you cut along its diagonals u get 6 identical pyramids with height half the base.
