-
Finished your HSC? Check out our University Discussion, Non-School forums or help out next year's HSC students! -
YOU can help the next generation of students in the community! Share your trial papers and notes on our Notes & Resources page
recurrence relationship q (1 Viewer)
- Thread starter =)(=
- Start date
5uckerberg
Well-Known Member
- Joined
- Oct 15, 2021
- Messages
- 562
- Gender
- Male
- HSC
- 2018
Lets do this the easy way shall we.
First, start with
This type of question the second part in particular are rare but are one of those "All roads lead to Rome but some are messier than others."
Last edited:
ExtremelyBoredUser
Bored Uni Student
wdym mx2 hes gonna destroy uni at this rate lmao
5uckerberg
Well-Known Member
- Joined
- Oct 15, 2021
- Messages
- 562
- Gender
- Male
- HSC
- 2018
Be careful of making such prophesies for one needs to let the person learn at their own rate and not be pressured unfairly. What you say might not occur and he might burn out if he does not know what he is doing.
Wise words
Aww thank you guys
ExtremelyBoredUser
Bored Uni Student
not intended as a prophecy as implied from the casual tone/punctuation but more of a hyperbolic compliment to demonstrate my appreciation of his effort but I understand where you're coming from. If it was taken or subtly felt as I was expecting him to be the next euler, newton... then apologies for that.
Yeah its been a joy just seeing qs from you, regardless of however you perform you should be really proud of yourself for going above and beyond
Last edited:
5uckerberg
Well-Known Member
- Joined
- Oct 15, 2021
- Messages
- 562
- Gender
- Male
- HSC
- 2018
Well, that is fine. I am not getting mad, just a word of caution.
ExtremelyBoredUser
Bored Uni Student
Yeah ik just mb if it came off that way because that wasnt my intent
5uckerberg
Well-Known Member
- Joined
- Oct 15, 2021
- Messages
- 562
- Gender
- Male
- HSC
- 2018
Alternatively if you wanted do it the hard way...
using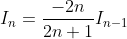
we can just continually evaluate 
to get
}{2(n-1)+1}\times\frac{-2(n-2)}{2(n-2)+1}\times...\times\frac{-2(2)}{2(2)+1}\times\frac{-2(1)}{2(1)+1}\times{I_{0}}\\\\\\I_n=\frac{-2n}{2n+1}\times\frac{-2(n-1)}{2(n-1)+1}\times\frac{-2(n-2)}{2(n-2)+1}\times...\times\frac{-2(2)}{2(2)+1}\times\frac{-2(1)}{2(1)+1}\times1\\\\\\I_{n}=\frac{(-1)^n\cdot2^n\cdot{n!}}{(2n+1)(2n-1)(2n-3)...5\cdot3\cdot1})
Looking at the denominator, it almost appears like (2n+1)! but it seems to be going down by 2s skipping all the even numbers. So we can add the even numbers by multiplying the numeratpr and denominator by the 'missing' set of numbers, to get (2n+1)!
^n\cdot2^n\cdot{n!}\cdot(2n)(2n-2)(2n-4)...6\cdot4\cdot2}{(2n+1)(2n)(2n-1)(2n-2)(2n-3)...5\cdot4\cdot3\cdot2\cdot1}\\\\\\I_{n}=\frac{(-1)^n\cdot2^n\cdot{n!}\cdot2^n\cdot{n}(n-1)(n-2)...3\cdot2\cdot1}{(2n+1)!}\\\\\\I_{n}=\frac{(-1)^n\cdot2^{2n}\cdot{n!}\cdot{n!}}{(2n+1)!}\\\\\\I_{n}=\frac{(-1)^n\cdot2^{2n}\cdot{(n!)^2}}{(2n+1)!}\ \text{as required})
using
Looking at the denominator, it almost appears like (2n+1)! but it seems to be going down by 2s skipping all the even numbers. So we can add the even numbers by multiplying the numeratpr and denominator by the 'missing' set of numbers, to get (2n+1)!
5uckerberg
Well-Known Member
- Joined
- Oct 15, 2021
- Messages
- 562
- Gender
- Male
- HSC
- 2018
Oh you are going to have to do a lot of integration by parts for thatAlternatively if you wanted do it the hard way...
using
Looking at the denominator, it almost appears like (2n+1)! but it seems to be going down by 2s skipping all the even numbers. So we can add the even numbers by multiplying the numeratpr and denominator by the 'missing' set of numbers, to get (2n+1)!
