Drsoccerball
Well-Known Member
- Joined
- May 28, 2014
- Messages
- 3,650
- Gender
- Undisclosed
- HSC
- 2015
Thanks mainly Integrand for saving me from dropping out of my degree by helping me in maths 1B but I need everyone's help just a bit longer for discrete maths.
 For B $ = $ { $ \varnothing,0,{1} $ } , are the following statements true or false? $ )
 \varnothing \subset $ B. Why ?$ )
 {\varnothing} \in $ B. Why ?$ )
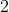 You can give a one word answer for this: Does A x B = all possible subsets of combinations of 1 element of A and 1 element of B? $ )
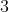 Does the fundamental theorem of arithmetic also deduce that two numbers when written as a product of their primes if different then the original numbers are different? Eg: $ n_1 = p_1^{\alpha_1}p^_2{\alpha_2}...p_n^{\alpha_n} $ and $ n_2 = q_1^{\beta_1}q_2^{\beta_2}...q_n^{\beta_n} \therefore n_1 \neq n_2 $ Since $ n_1 $ and $ n_2 $ have different prime factorizations.? $)
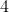 a,b,q and r are integers such that $ a= qb + r $ where $ a, b $ are non-zero. Prove that $ gcd(a,b) = gcd(b,r) )
Last edited:



