-
Want to level up your HSC prep on those harder Maths questions? Register now for the BoS Trials (7th October)!
Make a Difference – Donate to Bored of Studies!
Students helping students, join us in improving Bored of Studies by donating and supporting future students!
HSC 2012 MX1 Marathon #1 (archive) (1 Viewer)
- Thread starter nightweaver066
- Start date
Re: 2012 HSC MX1 Marathon
Yes, yes it would :\wouldnt it be sqroot(16-t^2)/4
kingkong123
Member
- Joined
- Dec 20, 2011
- Messages
- 98
- Gender
- Male
- HSC
- 2012
Re: 2012 HSC MX1 Marathon
the answer is 19. i got it by expanding and it didn't take as long as i expected, but is there a way of doing it without expansion? using binomial theorem or something?
Octagon
New Member
- Joined
- Jan 29, 2012
- Messages
- 22
- Gender
- Male
- HSC
- 2012
Re: 2012 HSC MX1 Marathon
Trig Help
A vertical tower of height h meters stands at the top of a slope of inclination A to the horizontal. An observer at the foot of the slope finds the angle of elevation of the top of the tower to be B. The observer walks a distance d m up a line of greatest slope, towards the foot of the tower, and finds the angle of elevation of the top of the tower to be Y. If the observer is then x m away from the foot of the tower, prove that
a. h=dsin(B-A)sin(Y-A)/sin(Y-B)cosA
b. x=dsin(B-A)cosY/sin(Y-B)cosA
Trig Help
A vertical tower of height h meters stands at the top of a slope of inclination A to the horizontal. An observer at the foot of the slope finds the angle of elevation of the top of the tower to be B. The observer walks a distance d m up a line of greatest slope, towards the foot of the tower, and finds the angle of elevation of the top of the tower to be Y. If the observer is then x m away from the foot of the tower, prove that
a. h=dsin(B-A)sin(Y-A)/sin(Y-B)cosA
b. x=dsin(B-A)cosY/sin(Y-B)cosA
bleakarcher
Active Member
- Joined
- Jul 8, 2011
- Messages
- 1,503
- Gender
- Male
- HSC
- 2013
Re: 2012 HSC MX1 Marathon
(x+1+x^(-1))^4=(x+1+x^(-1))^2(x+1+x^(-1))^2=[(x+1)^2+2x^(-1)(x+1)+x^(-2)](x+1+x^(-1))^2=[x^2+2x+2x^(-1)+x^(-2)+3][x^2+2x+2x^(-1)+x^(-2)+3]
Hence, constant term=1*1+2*2+2*2+1*1+3*3=19
Yeh, sorry made a mistake yesterday. You dont have to expand to whole thing you only have to expand part of it then analyse to find the constant term.the answer is 19. i got it by expanding and it didn't take as long as i expected, but is there a way of doing it without expansion? using binomial theorem or something?
(x+1+x^(-1))^4=(x+1+x^(-1))^2(x+1+x^(-1))^2=[(x+1)^2+2x^(-1)(x+1)+x^(-2)](x+1+x^(-1))^2=[x^2+2x+2x^(-1)+x^(-2)+3][x^2+2x+2x^(-1)+x^(-2)+3]
Hence, constant term=1*1+2*2+2*2+1*1+3*3=19
nightweaver066
Well-Known Member
- Joined
- Jul 7, 2010
- Messages
- 1,585
- Gender
- Male
- HSC
- 2012
Re: 2012 HSC MX1 Marathon
Use the method of bisection to solve to 2 decimal places:
 = e^x - 2)
Use the method of bisection to solve to 2 decimal places:
Re: 2012 HSC MX1 Marathon
I think you might be able to complete the square on x+x^(-1), but I haven't tried that yet...Yeh, sorry made a mistake yesterday. You dont have to expand to whole thing you only have to expand part of it then analyse to find the constant term.
(x+1+x^(-1))^4=(x+1+x^(-1))^2(x+1+x^(-1))^2=[(x+1)^2+2x^(-1)(x+1)+x^(-2)](x+1+x^(-1))^2=[x^2+2x+2x^(-1)+x^(-2)+3][x^2+2x+2x^(-1)+x^(-2)+3]
Hence, constant term=1*1+2*2+2*2+1*1+3*3=19
deswa1
Well-Known Member
- Joined
- Jul 12, 2011
- Messages
- 2,251
- Gender
- Male
- HSC
- 2012
Re: 2012 HSC MX1 Marathon
This is an interesting question:
Consider a circle with equation <a href="http://www.codecogs.com/eqnedit.php?latex=x^2@plus;y^2=10" target="_blank"><img src="http://latex.codecogs.com/gif.latex?x^2+y^2=10" title="x^2+y^2=10" /></a>. From the point P (-3,4), two tangents to the circle are drawn. Find the angle between these two tangents.
This is an interesting question:
Consider a circle with equation <a href="http://www.codecogs.com/eqnedit.php?latex=x^2@plus;y^2=10" target="_blank"><img src="http://latex.codecogs.com/gif.latex?x^2+y^2=10" title="x^2+y^2=10" /></a>. From the point P (-3,4), two tangents to the circle are drawn. Find the angle between these two tangents.
Re: 2012 HSC MX1 Marathon

Is answer ?:This is an interesting question:
Consider a circle with equation <a href="http://www.codecogs.com/eqnedit.php?latex=x^2@plus;y^2=10" target="_blank"><img src="http://latex.codecogs.com/gif.latex?x^2+y^2=10" title="x^2+y^2=10" /></a>. From the point P (-3,4), two tangents to the circle are drawn. Find the angle between these two tangents.
Last edited:
deswa1
Well-Known Member
- Joined
- Jul 12, 2011
- Messages
- 2,251
- Gender
- Male
- HSC
- 2012
Re: 2012 HSC MX1 Marathon

Yep. That's what me and a few friends got but we don't have the actual answer so I can't tell you 100%. I'm pretty confident though. How did you get it so fast, my method was quite complicated... If you don't want to type it all out, can you just post a general outline of your workingIs answer ?:
Carrotsticks
Retired
- Joined
- Jun 29, 2009
- Messages
- 9,467
- Gender
- Undisclosed
- HSC
- N/A
Re: 2012 HSC MX1 Marathon
Because Drongoski is brilliant.How did you get it so fast
Re: 2012 HSC MX1 Marathon
I got the answer in 1 minute. Unfortunately I'm hopeless in putting up a diagram. But I'll explain.
Circle is centered at origin with radius sqrt(10) and the external point (-3,4) has distance of 5 from the centre.{3,4,5} is a Pythagorean triple - so the 5 is immediate. Drawing the 2 tangents to the circle: consider 1 of the 2 congruent right-angled triangles: hypotenuse 5 and one of the shorter sides = sqrt(10). By Pythag Thm, remaining side is sqrt(15). Therefore half the required angle has tan = sqrt(10)/sqrt(15). Hence answer.
I got the answer in 1 minute. Unfortunately I'm hopeless in putting up a diagram. But I'll explain.
Circle is centered at origin with radius sqrt(10) and the external point (-3,4) has distance of 5 from the centre.{3,4,5} is a Pythagorean triple - so the 5 is immediate. Drawing the 2 tangents to the circle: consider 1 of the 2 congruent right-angled triangles: hypotenuse 5 and one of the shorter sides = sqrt(10). By Pythag Thm, remaining side is sqrt(15). Therefore half the required angle has tan = sqrt(10)/sqrt(15). Hence answer.
SpiralFlex
Well-Known Member
- Joined
- Dec 18, 2010
- Messages
- 6,954
- Gender
- Female
- HSC
- N/A
Re: 2012 HSC MX1 Marathon
Let

Substitute mx+c into the equation, and for intersections delta = 0
Substitute c as to your resultant equation.
Then use the identity
^2=(m_{1}+m_{2})^2-4m_{1}m_{2})
Then use the angle between two lines form.
This way is the fast way. For tedious and more elegant expansions,I got the answer in 1 minute. Unfortunately I'm hopeless in putting up a diagram. But I'll explain.
Circle is centered at origin with radius sqrt(10) and the external point (-3,4) has distance of 5 from the centre.{3,4,5} is a Pythagorean triple - so the 5 is immediate. Drawing the 2 tangents to the circle: consider 1 of the 2 congruent right-angled triangles: hypotenuse 5 and one of the shorter sides = sqrt(10). By Pythag Thm, remaining side is sqrt(15). Therefore half the required angle has tan = sqrt(10)/sqrt(15). Hence answer.
Let
Substitute mx+c into the equation, and for intersections delta = 0
Substitute c as to your resultant equation.
Then use the identity
Then use the angle between two lines form.
deswa1
Well-Known Member
- Joined
- Jul 12, 2011
- Messages
- 2,251
- Gender
- Male
- HSC
- 2012
Re: 2012 HSC MX1 Marathon
 . I'm going to rep you for that.
. I'm going to rep you for that.
That is fantasticI got the answer in 1 minute. Unfortunately I'm hopeless in putting up a diagram. But I'll explain.
Circle is centered at origin with radius sqrt(10) and the external point (-3,4) has distance of 5 from the centre.{3,4,5} is a Pythagorean triple - so the 5 is immediate. Drawing the 2 tangents to the circle: consider 1 of the 2 congruent right-angled triangles: hypotenuse 5 and one of the shorter sides = sqrt(10). By Pythag Thm, remaining side is sqrt(15). Therefore half the required angle has tan = sqrt(10)/sqrt(15). Hence answer.
Carrotsticks
Retired
- Joined
- Jun 29, 2009
- Messages
- 9,467
- Gender
- Undisclosed
- HSC
- N/A
Re: 2012 HSC MX1 Marathon
Unless you have trouble with that as well.
You have no choice but to use the usual techniques ie: differentiate, let it = 0 for SP, let double derivative be 0 for POI etc etc.I'm terrible at graphing so could someone help me out with how to tackle this curve?
y = x^4 - 6x^2 + 8x +1
Thank you so much!!
Unless you have trouble with that as well.
Re: 2012 HSC MX1 Marathon
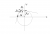
This is what Drongoski's diagram would look like:I got the answer in 1 minute. Unfortunately I'm hopeless in putting up a diagram. But I'll explain.
Circle is centered at origin with radius sqrt(10) and the external point (-3,4) has distance of 5 from the centre.{3,4,5} is a Pythagorean triple - so the 5 is immediate. Drawing the 2 tangents to the circle: consider 1 of the 2 congruent right-angled triangles: hypotenuse 5 and one of the shorter sides = sqrt(10). By Pythag Thm, remaining side is sqrt(15). Therefore half the required angle has tan = sqrt(10)/sqrt(15). Hence answer.
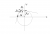
Re: 2012 HSC MX1 Marathon
Thanks man. That's the diagram I would have loved to provide.This is what Drongoski's diagram would look like:
View attachment 24465
Last edited:
Carrotsticks
Retired
- Joined
- Jun 29, 2009
- Messages
- 9,467
- Gender
- Undisclosed
- HSC
- N/A
Re: 2012 HSC MX1 Marathon
a) Differentiate it and show that y' = 0 has no solutions
b) Let f''(x) = 0 and solve for x.
a) Differentiate it and show that y' = 0 has no solutions
b) Let f''(x) = 0 and solve for x.

