Re: HSC 2013 4U Marathon
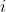$ \sum_{r=0}^{n}(r+1)^k - r^k = (n+1)^k)
by a telescoping sum.
Now expanding the binomial first instead of using a telescoping sum gives:
^k = \binom{k}{0} + \binom{k}{1}r + \binom{k}{2}r^2 + ... + \binom{k}{k}r^k)
Now if we apply the summation, we sum each one individually, that is:
^k = \sum_{r=0}^{n}\binom{k}{0} + \sum_{r=0}^{n}\binom{k}{1}r + ... + \sum_{r=0}^{n}\binom{k}{k}r^k)
Now using our definition of
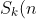)
we obtain:
^k = (n+1) + \binom{k}{1}S_1(n) + \binom{k}{2}S_2(n) + ... + \binom{k}{k-1}S_{k-1}(n) + \binom{k}{k}S_k(n))
Using the symmetry property of binomial expansions gives:
^k = (n+1) +\binom{k}{k-1}S_1(n) + \binom{k}{k-2}S_2(n) + ... + \binom{k}{1}S_{k-1}(n) + \binom{k}{0}S_k(n))
Now since
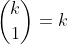
and
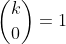
we get:
^k = (n+1) +\binom{k}{k-1}S_1(n) + \binom{k}{k-2}S_2(n) + ... + kS_{k-1}(n) + S_k(n))
Now consider the second part of the equation:
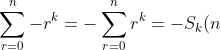)
by definition.
Hence if we add the two parts together we obtain:
^k - r^k = (n+1) +\binom{k}{k-1}S_1(n) + \binom{k}{k-2}S_2(n) + ... + kS_{k-1}(n) + S_k(n) - S_k(n))
So the two terms on the end cancel out and give:
^k - r^k = (n+1) +\binom{k}{k-1}S_1(n) + \binom{k}{k-2}S_2(n) + ... + kS_{k-1}(n))
But this was equal to the value of the telescoping sum, so we can equate the two and get:
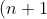^k = \sum_{r=0}^{n}(r+1)^k - r^k = (n+1) +\binom{k}{k-1}S_1(n) + \binom{k}{k-2}S_2(n) + ... + kS_{k-1}(n))
Taking everything from the RHS to the LHS except for the last term gives us the required result of:
 = (n+1)^k - (n+1) - \binom{k}{k-1}S_1(n) - \binom{k}{k-2}S_2(n) - ... - \binom{k}{2}S_{k-2}(n))
Which can be re-written in the form asked:
 = (n+1)^k - (n+1) - \binom{k}{2}S_{k-2}(n) - \binom{k}{3}S_{k-3}(n) - ... - \binom{k}{k-1}S_1(n))
Quod Erat Demonstrandum.
)
 + nsin(\theta)[1-sin(n\theta)]}{2-2cos\theta} = \sum_{k=1}^{n} ksin(k\theta))

