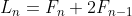Carrotsticks
Retired
- Joined
- Jun 29, 2009
- Messages
- 9,467
- Gender
- Undisclosed
- HSC
- N/A
Re: 2012 HSC MX2 Marathon
Very nice question.Here's an interesting question I just did:
Generate a formula for the nth term of the Fibonacci series (1,1,2,3...)
Hence, find a formula for the nth term of the Lucas series (1,3,4,7...)given that